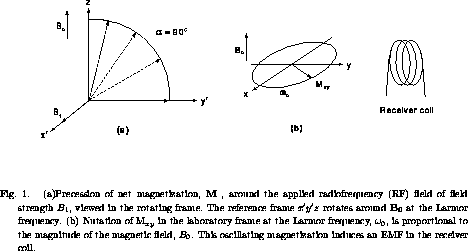Basic physics

MR physics: Basic physics - the MR signal

In almost all MRI applications, the signal originates from the hydrogen nucleus, which is a single proton. The relevant property of the proton is its spin ; a simple classical picture of spin is a charge distribution in the nucleus rotating around an axis collinear with . The resulting current has an associated dipole magnetic moment 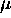, collinear with 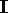; the quantum mechanical relationship between the two is where h is Planck's constant and 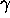is the gyromagnetic ratio. For protons, 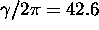MHz/T.

In a single volume element corresponding to a pixel in an MR image, there are many protons, each with an associated dipole magnetic moment, and the net magnetization, 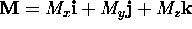of the volume element is the vector sum of the individual dipole moments, where i, j and k are unit vectors along the x, y, and z axes respectively. In the absence of a magnetic field, the spatial orientation of each dipole moment is random and .

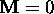This situation is changed by a static magnetic field 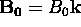. This field induces magnetic moments to align themselves in its direction, partially overcoming thermal randomization so that, in equilibrium, the net magnetization 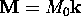represents a small fraction (determined from the Boltzmann distribution) of 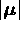times the total number of protons. Under typical imaging conditions ( T - approximately 20,000 times the Earth's magnetic field strength, and temperature near 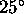C), the magnitude of the equilibrium magnetization, , roughly corresponds to 1 in 10 of the dipole moments in the volume being aligned with the field while the rest take on random orientations. While the fraction is small, the total number of contributing protons is very large, approximately 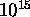dipoles in a 5 mm volume.

Equilibrium is not achieved instantaneously. Rather, from the time the static field is turned on, 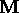grows from zero toward its equilibrium value along the z axis; that is,

where T1 is the longitudinal relaxation time. This equation expresses the dynamical behavior of the component of the net magnetization 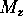along the longitudinal (z) axis.

The component of the net magnetization, 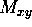, which lies in the transverse plane orthogonal to the longitudinal axis undergoes completely different dynamics. 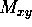, often referred to as the transverse magnetization, can be described by a complex quantity 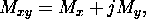where . This component precesses about , i.e., 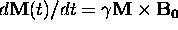. The precession frequency

is proportional to and is referred to as the Larmor frequency (Fig. 1b). This relation holds at the level of individual dipoles as well, so that .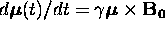

Accompanying any rotating dipole magnetic moment is a radiated electromagnetic signal circularly polarized about the axis of precession; this is the signal detected in MRI. The usual receiver is a coil, resonant at , whose axis lies in the transverse plane - as 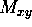precesses, it induces an emf in the coil.

A final question remains: if 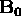induces a collinear equilibrium magnetization 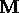, how can we produce precessing magnetization orthogonal to 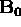? The answer is to apply a second, time-varying magnetic field which lies in the plane transverse to 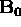. Let this field be described by 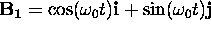. This field rotates about the static field direction 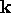at radian frequency 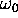. If we then place ourselves in a frame of reference (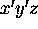) which also rotates at radian frequency 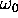, this second field appears stationary. Moreover, any magnetization component orthogonal to 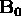no longer appears to rotate about . Instead, in this rotating frame, 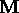appears to precess about the `stationary' field 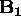alone with radian frequency . One can therefore choose the duration of 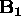, , 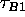so that 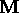is rotated into the transverse plane (Fig. 1b). 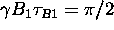The corresponding waveform is called a 90 excitation pulse ( radians).

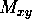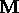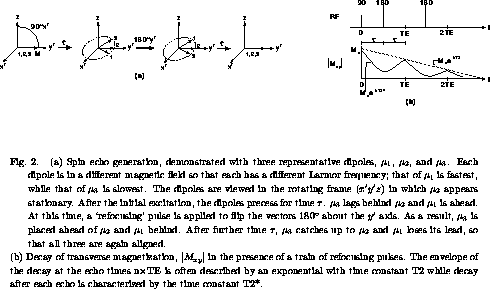
The signal from will eventually decay. Part of this decay is the result of the drive to thermal equilibrium where is brought parallel to , as described earlier. However, this is generally a secondary contribution to the decay rate. Recall that the individual dipole moments contributing to each precesses at a rate proportional to the magnetic field they experience. Since even a single volume element contains a heterogeneous physical environment, the magnetic field varies slightly among its protons and the phase coherence present immediately after the 90 pulse is gradually lost as the dipole moments contributing to 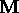precess at slightly different rates (Fig. 2a). Over time, the vector sum, 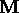, decreases in magnitude since the individual dipole moments no longer add constructively. The associated decay is characterized by an exponential with time constant T2 (Fig. 2b).

Hahn [5] discovered that this loss of transverse magnetization due to dephasing can be recovered to some extent by inducing a spin echo (Fig. 2). Specifically, let the dipole moments evolve for a time 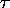after excitation. At this time, apply another